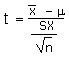
Eight students in PE 101j Joggling spring 2003 were asked to strongly disagree, disagree, agree, or strongly agree to the following statement, "I enjoyed participating in the physical activities of this class." The five responses were assigned numberical weights as seen below:
Strongly disagree: -2 | Disagree: -1 | Maybe: 0 | Agree: 1 | Strongly agree: 2
The eight answers from spring 2003 were 1, 1, 2, 1, 2, 2, 2, 2.
Use these eight spring 2003 answers to calculate the population values in your hypothesis test. This spring, spring 2004, the students were asked the same question again. This spring the average was 1.40. At an alpha of 0.10, is the spring 2004 average statistically significantly different than the spring 2003 average? Use a two-tailed test.
| Statistic or Parameter | Symbol | Equations | Excel | Hypothesis Testing |
|---|---|---|---|
| Degrees of freedom | df | = n-1 | =COUNT(data)-1 |
| Calculate a t-statistic (t) | t | 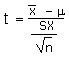 |
(x - µ)/(sx/sqrt(n)) |
| Calculate t-critical for a two-tailed test | tc | =TINV(a,df) | |
| Calculate a p-value from a t-statistic t | p | = TDIST(ABS(t),df,#tails) |