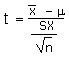
During the period 1971 to 2002 there was a sample size n = 31 named tropical storms and typhoons with a sample mean was x = 18.4 with a standard deviation of sx = 3.4. Calculate a 95% confidence interval for the expected range of the population mean µ based on the data using the student's t-distribution.
| Statistic or Parameter | Symbol | Equations | Excel |
|---|---|---|---|
| Find a tc value from a confidence level c and sample size n | tc | =TINV(1-c,n-1) | |
| Calculate an error tolerance E of a mean for any n ≥ 5 using sx. | E | =tc*sx/SQRT(n) | |
| Calculate a confidence interval for a population mean µ from a sample mean x and an error tolerance E | x-E≤ µ ≤x+E | ||
| Statistic or Parameter | Symbol | Equations | Excel |
| Hypothesis Testing | |||
| Degrees of freedom | df | = n-1 | =COUNT(data)-1 |
| Calculate a t-statistic (t) | t | 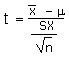 |
(x - µ)/(sx/sqrt(n)) |
| Calculate t-critical for a two-tailed test | tc | =TINV(α,df) | |
| Calculate a p-value from a t-statistic t | p | = TDIST(ABS(t),df,#tails) | |
| Calculate a maximum possible level of confidence c from a p-value (two-tailed) | max c = 1-p | ||